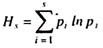In oxic bioprocess engineering system in liquid culture transfer of oxygen from air to liquid has been measured by fast response steam sterilizable dissolved oxygen probe on line.
In the measurement, the concept of flow of oxygen from higher concentration (heaviside) to lower concentration has been conceived.
1. Stagnant Film Concept:
Whitman based his film theory on Fick’s first law. In this concept, oxygen mass transfer occurred by molecular diffusion through a thin film in stagnant fluid underlying the surface of the bulk liquid phase. His theory is shown as,
(KL)w = D/h
D is the diffusivity of oxygen in the fluid/liquid.
2. Penetration Concept:
The assumptions made by Whitman in proposing his theory were modified by Higbie in his penetration theory. He assumed that contact between gas and liquid occurs in a series of intermittent steps, and the length of time (tc) that each element remains in contact with the gas is constant. On the basis of these assumptions, Higbie showed that
Where tc is the time of contact between gas and liquid in hours.
3. Surface Renewal Concept:
Danckwerts proposed a modification to the penetration theory of Higbie. It did not require the assumption of constant time of contact between the gas and elements of the liquid surface, and it considered continuous surface renewal of gas in the liquid. According to his surface renewal theory,
(KL)/D = √DS (5.17)
Where S is the fractional rate of surface renewal per hour
Kishenevsky, in a critical appraisal of the theoretical work of Danckwerts in the field of gas-liquid absorption, noted several inconsistencies in the surface renewal theory. Therefore, he concluded that Danckwert’s quantitative theory, based on the concept of molecular diffusion mechanism of mass transfer during the period of surface layer renewal, was contrary to the experimental data.
This divergence of opinion concerning the physical meaning of KL led to extensive studies on the oxygen mass transfer coefficient in gas-liquid absorbers. In a conventional air sparged and stirred bio-reaction system, it is difficult to study KL independent of the area term a, because the gas-liquid interfacial area available for oxygen mass transfer cannot be determined with any degree of accuracy.
Because of this difficulty, it was necessary to design a bioreactor system with a defined total surface area, through which gas-liquid transfer could occur and in which the dependence of KL on hydrodynamic conditions could be studied by varying liquid flow or stirring intensity in absorption by the liquid film having a large surface area.
4. Simultaneous Adsorption-Absorption Concept:
In discussing controlling factors in liquid film absorption, a hypothetical case has been proposed in which the gas was completely insoluble in the liquid and could be adsorbed only on the surface. For such a case, the transfer rate of the gas would depend only on the rate of new surface generation instead of diffusion through a liquid film.
It was proposition by Hartman that, in all probability, without adsorption, no absorption would occur, although adsorption might occur without absorption. It was also stated that before absorption can take place, the surface must be wetted by the substance to be absorbed.
In order to wet the surface by the substance, it must be adsorbed first on the surface. To verify this, Phillips conducted experiments in the horizontal rotary thin-film bioreactor (HRTFB) of 3.8 cm diameter and 92 cm long using Candida utilis fermentation at 0.95 and 1.95 atm pressures. The results are shown in Figure 5.10.
Analysis of these results showed that the theoretically calculated amount of air that could be accommodated on 1 cm2 surface was 9.7 x 10-7 mmol, which is in close conformity with the reported values with other gases. Considering that air contains 21% oxygen, the amount of oxygen that would be carried into the liquid when rotating surface liquid film was submerged would be theoretically 2.04 x 10-7 mmol/cm2. This value compares most favorably with the value 2.0 x 10-7 mmol/cm2 determined from the experimental results of Phillips (Fig. 5.10) as mentioned above. Based on these reports and his findings, Phillips proposed a modified oxygen transfer rate equation in the liquid as
Here, the first term contributes for absorption and the second term for adsorption of oxygen in the liquid medium. Af and Y are indicating the rate of surface formation or submergence (cm2 h-1) and volumetric or mole fraction in the gaseous phase of gas being absorbed. Thus, the above equation may be referred to in defining the simultaneous adsorption-absorption concept.
5. Comparison of Concepts:
If it is assumed that the rate of fraction of surface renewal is equivalent to the reciprocal of contact time KL in Higbie’s concept, then the ratio applies.
Thus, the value of Danckwerts (KL)D is 1.13 times as large as Higbie’s (KL)H. A comparison of values of KL in different concepts is shown in Fig. 5.11. It should be noted that both the Higbie and the Danckwerts theories predicted that KL should vary nonlinearly with the time of contact or fractional rate of surface renewal. The validity of the same could be checked by Phillips, as shown in Fig. 5.11.
However, in the case of HRTFB, the relation was linear for surface renewal over a wide range of values. Shake flasks and un-baffled stirred vessels with surface aeration were not suitable for such a study, since the surface area in either system was difficult to define and varied as the shaker or stirrer/agitator speed was changed. In addition, the surface-to-volume ratio was unfavorable for obtaining high oxygen transfer rates.
Wetted-wall columns have been used for gas-liquid transfer studies. However, the rate of flow down or up the wetted wall is limited by gravitational force, and the requirement for recirculation of the fermentation liquid dictated an aseptic pumping operation.
Heaviside Theory (HST) based Concept:
In oxic bioprocess engineering system in liquid culture transfer of oxygen from air to liquid has been measured by fast response steam sterilizable dissolved oxygen probe on line. In the measurement, the concept of flow of oxygen from higher concentration (heaviside) to lower concentration has been conceived.
Thus, heaviside theory (HT) in oxygen transfer is of significance. The first aim of the present work is to make a mathematical foundation of HT pertaining to simple air-liquid contacting system that prevails in bio-liquids. With this primary objective we have considered unsteady state mass transfer of oxygen into aqueous water. Thus, our starting point was the second law of diffusion equation,
6. Additional Information:
Revolving drums equipped with internal baffles and buckets or concentric horizontal rotary cylinders with spargers were used in the microbial production of gluconic acid. Various types of horizontal thin-film rotary bioreactors/fermentors have been used with diverse objectives (Fig. 5.12 and 5.13).
The rotational speed of these drums/cylinders was limited to only 13-20 rpm because of foam formation caused when the liquid was aerated. It was reported that at very low rotational speeds of this type of gas-liquid contactor, KLa was found to decrease appreciably. At lower speeds, surface renewal rates of the liquid film around the inner periphery of HRTFB are slow, so a low KLa results (Fig. 5.14).
At high rotation speeds, oscillation of the bulk liquid inside the vessel may occur because of liquid drag. However, in the fermentations thus far investigated in HRTFB, low rotational speeds have been used. At such speeds, oscillation of bulk liquid does not appear because of low drag force.
When air is allowed to flow through an HRTFB, oxygen is mainly absorbed in the thin film of liquid formed on the peripheral surface when the rotational speed is low with no oscillation in bulk liquid.
Oxygen transfer behavior of the system can be analyzed with the assumptions that:
(1) There is negligible change in the specific surface area of oxygen transfer due to an increase in rotation speed;
(2) Liquid film thickness is uniform and small, so that the distance from the center of the vessel to the film surface is approximately equal to the radius of the vessel; and
(3) The angle formed by the film surface edges with the center of the vessel is constant under nonoscillating conditions of the bulk liquid.
Under these conditions, the specific liquid film surface renewal per unit contact time with the gas can be written as
Where
a’ is specific liquid film surface renewal (cm2/cc);
tc is contact time between liquid film surface and gas (min);
r is distance of the liquid film from the center of the vessel = radius of the vessel (cm);
1 is longitudinal length of the liquid film (cm);
v is volume of the culture liquid in the fermenter, (cc);
Ө is angle formed by the film surface and the center of the vessel, (deg); and
N is rotational speed of the fermenter (min-1).
Therefore, from the equation above, the fraction surface renewal rate is
Since the renewal of the liquid film surface exposed to gas is continuous, Danckwerts’ surface renewal theory is applied to obtain the liquid film transfer coefficient KL as:
Since during fermentation the physical environment remains unchanged, D may be assumed constant, thus,
KL α N 1/2 (5.34)
This means that with a given surface area in the HRTFB, KL is directly proportional to the square root of the rotation speed of the vessel. For verification of these relations, studies (Figs. 5.12 and 5.13) were carried out with different microbial systems at five rotational speeds of HRTFB. The values of KLa were determined at each speed. No oscillation of the bulk liquid was observed in the range of rpm employed. A plot of KLa as a function of rotation speed is shown in Fig. 5.14.
The value of the liquid film surface area of oxygen transfer a and the angle 0 formed by the liquid film surface edges with the center of the vessel were computed from the vessel’s geometry. From the known values of Ө, the contact time tc between the gas and the liquid film surface areas, and fractional surface renewal rates, 5 could be determined from the above equations easily for each rotation speed, N. The variation of tc and S as functions of N is shown in Fig. 5.15.
From the measured value of KLa and the known value of a versus N plot (Fig. 5.15) indicated a linear relationship according to equation 5.34. It follows that the rotational speed of an HRTFB changes only as a square of KL, provided the hydrodynamic conditions remain unaltered. The positive intercept on the ordinate as noticed at zero rotation speed (Fig. 5.16) represents the net transfer of oxygen through a still surface having a total area equivalent to the wetted surface exposed to air when the fermenter/ bioreactor was not rotated.
In a horizontal rotary thin-film bioreactor, transport of oxygen from gas phase to liquid depends on rotation speed or peripheral velocity (VP), thickness of the liquid film (hf) on the rotating surface, and hydrodynamics of physical properties of liquid containing respiring microbial cells. After sparging of air in the liquid pool in the HRTFB through a longitudinal sparger, the transport of oxygen from air into the liquid takes place mostly through the rotating liquid film having a large surface area, and a small fraction of it is absorbed due to sparging. Thus, equation 5.34 can be written in rate equation form as:
Where KLa0 is overall volumetric oxygen mass transfer coefficient during sparging, rX is the oxygen uptake rate in which r is the specific oxygen uptake rate and X is the cell mass concentration. In HRTFB actually,
where KLaf and KLas are the values of volumetric oxygen transfer coefficient in the rotating liquid film, and due to sparging in the liquid pool without rotation, respectively. KLa0 and KLas are determined by the standard method. From the difference of the known values of KLa0 and KLas, the value of can be obtained.
The value of KLaf is also computable as below.
To check this relation, the film thickness of liquid (hf) on the rotating surface is computed from the following correlation:
Here μam is the value of maximum apparent viscosity, pa is apparent density, and σa is value of surface tension, K is a constant, and g is acceleration due to gravity. In order to check the validity of the correlation experiments with Trichoderma reesei, QM9414 cell cultivations were conducted at different rotation speeds in a 60-liter HRTFB at 30°C for 160h. In the cultivation broth, typical dissolved oxygen traces obtained – by the gassing out method with/without rotation of the HRTFB were as given in Fig. 5.17.
Evaluation of KLa0, and KLas from these profiles at different rotation speeds of HRTFB showed values listed in Table 5.1, which indicated that with no rotation the value of KLas is very small. In the used rotation speed range, KLa0 and KLaf increased with the increase in reactor rotation speed.
At a given Vp, when the maximum respiration of cell culture was observed, maximum cell growth rate occurred. At this state, the values of (ʯam, pa, and oa were measured in the cell culture liquid. Values of hf were computed and are shown in Table 5.1, from which it was seen that the computed values of √N/hf had a correlation with KLaf as shown in Fig. 5.18, indicating inverse proportionality. The proportionality constant was VD0 / 360 whose value was obtained from the slope of Fig. 5.18.
From this slope, the value of D was found to be 0.20 x 10-5 cm2 s-1. This value of D is of the same order of diffusivity of oxygen in water (20°C), but the value is about 8-10 times less. The low value of D was perhaps due to the presence of solute content in the liquid.
From these results, it was concluded that in the transport of oxygen in the gas-liquid microbial system in HRTFB, liquid film thickness on the rotating surface has a great influence on the overall volumetric oxygen transfer coefficient. A large fraction of oxygen is absorbed in the liquid film having a large surface area.
In stirred tank reactor mixing/agitation become advantageous by:
1. Creating large air-liquid interfacial area by bubble disruption,
2. Reducing the thickness of the liquid film around the air bubble thus enhancing oxygen diffusion,
3. Maintaining homogeneity of dissolved oxygen (DO) in the liquid by reducing resistance to bulk mixing and
4. Controlling clump size, a phenomenon common with mould and actinomycetes, by reducing chances of agglomeration of cells thereby decreasing intra-clump resistance.