In this article we will discuss about the generation time of bacteria.
Most bacteria reproduce by binary fission, which results in doubling of the number of viable bacterial cells. Therefore, during active bacterial growth, the number of bacterial cells and, hence their population, continuously doubles at specific time intervals because each binary fission takes a specific duration of time.
This ‘specific time interval’ between two subsequent binary fissions is known as generation time or doubling time.
It we start with a single bacterial cell, its fission proceeds as a geometric progression (exponential growth) with one cell dividing to form two, these two to four, further to eight and so on i.e. each succeeding fission (generation), assuming no cell death, doubles the population size.
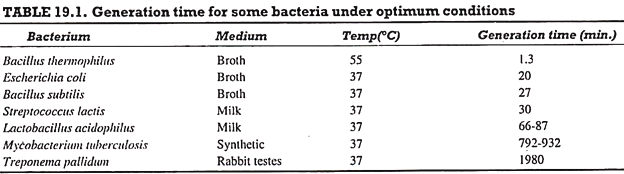
Generation time or doubling time varies considerably among different bacteria (Table 19.1). A bacterium such as E. coli enjoys generation time as short as 20 minutes under optimal conditions, although in nature many bacteria have generation times of several hours. One cell of E. coli with a 20 minute generation time, for convenience, will multiply to 512 cells in 3 hours, to 4096 cells in 4 hours, and to 32768 cells in 5 hours, and so on.
Mathematical Expression of Growth:
To calculate the generation time of individual microorganisms the following experimental data are required:
1. The number of organisms present at the beginning.
2. The number of organisms present at the end of a given time interval.
3. The time interval.
The relationship of cell numbers and generations can be expressed in a series of equations. Starting with a single cell, the total population B at the end of a given time period would be expressed as
B = 1 x 2n
Where 2n is the bacterial population after n generations. However, under practical conditions several thousands of bacteria are introduced into the medium at zero time and not one, so the formula now becomes.
Bn = B0 x 2n
Where B0 = number of organisms at zero time.
Bn = number of organisms after n generations.
n= number of generations.
Solving the equation for n, we have
log Bn = log B0 + n log 2
n = log Bn – log B0 /log2
Thus we can calculate the number of generations if we know the initial population B0 and the population Bn after time t. The generation time G is equal to t (the time which elapsed between B0 and Bn) divided by the number of generations n, or
G=t/n = t log2/ log Bn – log B0
An alternative method is used to describe bacterial growth in mathematical terms when the culture is undergoing balanced growth. The rate of increase in bacteria at any particular time is proportional to the Cell number of mass or bacteria present at that time (Fig. 19.1).
The constant of proportionality is an index of the rate of growth and is called the exponential growth rate constant (K). It is defined as number of doublings in unit time, and is usually expressed as the number of doubling in an hour.
It is calculated from the following equation:
Bn= B0 x 2Kt
Bn = Population at time t.
B0 = Population at time zero.
Taking the logarithms
log Bn = log B0 + Kt log 2, and
Solving the equation for K
K = log Bn – log B0 /t log 2
The exponential growth rate constant is therefore reciprocal to generation time, i.e.
G = 1/K
For example, generation time of E. coli is 20 minutes, i.e. 1/3 hour.
1/3 = 1/K
K = 3 doublings per hour.