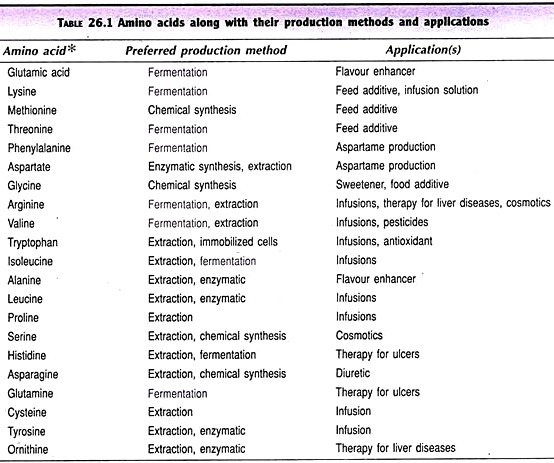
Each population is a separate entity showing several characteristics such as spacing, size, density, natality, mortality, age structure, growth, fluctuations and cycle.
1. Spacing:
The pattern of spacing of organisms is an important feature of every population.
Spacing may be uniform, random or clumped. Uniform spacing is shown by invertebrate populations; random pacing occurs among plants; clumped spacing may be shown by mating individuals.
Besides such clumping for reproduction, animals form swarms, herds, flocks or colonies for feeding and protection. According to Alice’s principle, each species has an optimum clunking value and overcrowding beyond this state or under crowding below it tends to act as a limiting factor in population regulation.
2. Size and Density:
The success of a population is reflected in its size and density. It is easy to define the population size when population consists of unitary organisms; it is simply the number of individuals present in a given area.
Population density is the number of individuals per unit area (or volume). The unit of area in measuring the density varies. This may be expressed, for example, the number of microorganisms per litre of water. For larger animals or plants, square meter, square km or acre may be the unit. The density of a population may be studied in several ways.
The abundance of s population in a given geographic region is termed the average or regional density. A region, however, usually includes unfavorable parts in which the individuals may be rare or absent, and r suitable niches in which the individuals occur in large number. The abundance of a population within its niche is called the economic or niche density, which is always higher than the regional density.
However, in many cases, it is impractical to determine the absolute density of a population and it may be easy to know the relative density of the population (i.e., the area x has more individuals than area y).
The density of a population may be almost constant, not increasing or decreasing at a given time. If the population density is increasing, this means that the natality (birth rate) is higher than the mortality (death rate).
If it is decreasing, then obviously the number of individuals in the population who are dying per unit time exceeds the birth rate, the number of individuals produce per unit time.
When birth rate and death rate are almost equal, the population density will appear to be constant for a given time. Thus, changes in population size over time can be calculated b adding new born individuals (B) and the number of immigrants (i) to the original population a time t (Nt) and subtracting the number of deaths (D) and emigrants (E) to give a new population size at the time t+1 (Nt+1).
This may be represented by the equation:
Nt+1 =Nt + B + I- D- E
According to krebs (1994), the four processes – natality, mortality, immigration, and emigration are the primary population parameters and affect the density as shown below:
The estimation of population density may be made by several methods. The use of quadrat is the simplest method. A quadrat is a sampling area of any shape, but a quadrat of square o rectangular shape is preferred. The size or area of the quadrat depends on the sampling need and may be 0.5 m2 or Im2.
The general procedure is to count all the individuals on several quadrate of known size and to extrapolate the average to the whole area. The use of quadrates is the most common method for sampling plants. The method has also been used for sampling animals sue as ants, earthworms and mollusks.
One of the recent and more widely used methods is CMR technique, i.e. capture a number of animals, tag or mark them in one fashion or another (e.g., by clipping their tails, piercing their ears, or attaching some marker), release them, and then at subsequent time obtain another sampling of animals. The proportion of the originally marked animals in the second sampling within the same area provides die basis for estimating the population density.
This method is known as the Petersen method. It is also called the Lincoln method by will life ecologists. Dahl (1919) used this method for estimating trout density in lakes.
3. Age Composition:
There are three major age groups in a population. These are pre reproductive, reproductive and post reproductive. These categories, proposed by Bodenheimer (1985), have considerable value in visualizing the status of a population. In natural population, the age structure is almost constantly changing. In a rapidly growing or expanding population as in the case of bacteria, yeast and housefly, birth rate is high and population growth may be exponential. Under these conditions each successive generation will be more numerous than the preceding one and, as a result, a pyramid structure results.
In a stable population, a bell-shaped age structure is obvious as the pre-productive and reproductive age groups become more or less equal in size and the post reproductive group remains the smallest. In a declining population, an um-shaped age structure is own (Fig. 3.1) indicating the smallest size of pre-reproductive category or young individuals.
However, we rarely find a natural population having a stable age structure or a stationary age distribution. In some long-lived species such as fish and trees, dominant year-classes may be In human population, differences in mortality rates have little effect on age composition, while changes in birth rates have a large effect on age structure (Barclay, 1958).
(a) Pyramid age structure,
(b) Bell-shaped age structure,
(c) Um-shaped age structure
4. Survivorship Curves:
In order to understand a survivorship curve we should first know what is a cohort? A cohort may be defined as a group of individuals of the same age recruited into a population at the same time. A cohort life table is also called the dynamic life table, which may be defined as the age- specific survival and fecundity of a cohort followed from birth to death of the last individual.
A survivorship curve is a plot of the declining size of a cohort as the individuals die usually with time on the horizontal axis and log population size (log10 Ix) on the vertical axis where Ix is the proportion of the original cohort still alive. A survivorship curve is a graph to show the number of individuals, which survive per thousand of population through each phase of life represented by age in years.
Another method of showing a survivorship curve is to use a log scale for the number of individuals. This type of semi-log plot indicates the population dynamics of the organism and is therefore quite useful. Fig. 3.2 shows three general shapes of survivorship curves on a semi-log y plot. These curves illustrate different patterns of population dynamics. (A) shows a steady mortality me during each phase of life; (B) shows that the highest mortality rate occurs m older individuals; (c) shows high mortality in the young with very little in adults.
However, natural populations rarely show extreme cases like (B) or (C) and they often show a wavy curve. The shape of the survivorship curves may be used to show the critical periods the life of an organism. As the curve becomes steeper there is an increase in mortality, which indicates some environmental or other effect on the population. The normal plot curve shows most clearly the early pattern of mortality in the young individuals, whereas the semi-log curve shows the pattern of mortality in the older individuals more clearly.
5. Population Growth:
Of the several dynamic features of a population, the most important is the growth, the capacity for increase in individual numbers. By measuring the size or density of a given population from time to time we can obtain important information regarding its rate of increase, and can also predict future changes in its size. To measure the growth of a population of a particular species in the laboratory, say bacteria or yeast cells, first we transfer a relatively small number of the given individuals to a flask containing appropriate nutrient solution, and then proceed to measure the population increase over a desirable period of few days.
Logistic Growth:
When a population is allowed to grow in a limited space (environment) it shows logistic growth. If we plot a graph of the number of bacteria or yeast cells against time, we find a typical S-shaped sigmoid curve called the population growth curve (Fig. 3.3), which usually consists of four phases. The first phase initially shows a slow rate of growth in population. This phase is often called the lag period, and is followed by a rapidly accelerating stage of multiplication.
These together constitute the first phase of population growth curve ,which is called the phase of accelerating multiplication. It is followed by a phase of extremely rapid population increase known as the logarithmic phase in which the number of individuals increase at an exponential rate. During the logarithmic phase, which represents the period of maximum increase in population, the number of organisms increases in a geometric fashion (2,4,8,16,32,64 and so on) instead of in arithmetic manner (2,4,6,8,10,12 and so on).
The logarithmic phase is succeeded by the phase of decelerating multiplication, during which the rate of population increase begins to slow down. The final phase, called the equilibrium phase, is one in which there is essentially no net change in the population since the birth rate more or less balanced by the death rate.
The S-shaped logistic growth curve is an adequate description for the laboratory population growth of organisms having simple life cycle such as Paramecium, yeast and the like. However, the population growth in organisms with more complex life cycle often does not follow the logistic growth curve. In particular, the stable asymptote of the logistic growth curve is not achieved in natural populations, but numbers fluctuate.
Although the total number of individuals and the time intervals are considerably different for organisms, the S-shaped curve is typical under optimal conditions of the growth of all populations of living organisms ranging from bacteria to man. However, the population growth curves of all organism fall off from the logarithmic phase to become ultimately the equilibrium phase instead of continuing unchecked. The reason is the environmental resistance comes to operate.
In other words, the various physical, chemical and biotic factors of the environment are incapable of supporting a maximal rate of reproduction for any organism over a long period of time. Such limitations as the restricted supply of nutrients, the inhibitory effects of accumulated metabolic waste products, and the hindrances imposed under natural conditions by competition, parasitism and predation markedly increase the death rate besides restraining the rate of reproduction.
The environment, therefore, is capable of supporting only a certain number of organisms of my given species, thus accounting for the inability of any population to maintain itself in the logarithmic phase for a long period of time. Thus, it becomes clear that there is no net change in the population when it is at equilibrium with the environment, i.e., it has reached the limit at which the environment can support the population. This limit is referred to as the carrying capacity of the environment. In simple words, carrying capacity is the number of individuals in a population which the environment (habitat) can support. It is represented by “K”.
Logistic Equation:
The logistic equation shows density-dependent growth. In other words, it describes the growth at a simple population in a limited space with limited resources.
The logistic equation may be written as:
dN/dt = rN (1-N/K)
dN/dt = rate of growth of population
r = intrinsic rate of increase (per individual of population)
N = population size (number of organisms in population at time t)
K = carrying capacity of population
(1 – N/K) = density – dependent factor
This equation predicts growth of a population over time to be sigmoidal, as is commonly observed in real population. Where N is very small, NIK is also very small and therefore (1 – NIK) 3 approximately 1 and the logistic equation becomes:
dNIdt = rN
As the carrying capacity is attained, N/K tends to 1 so the logistic equation becomes:
dN/dt = rN (1 – 1) = 0
In other words, the rate of population growth becomes zero.
Exponential Growth:
When the curve of population growth quickly begins to rise very steeply, the population shows exponential growth (Fig. 3.4), .K population growing exponentially increases according to the equation:
Nt = No ert
Geometric Growth:
Geometric growth (Fig. 3.5) may be defined as the population growth in which the rate of increase is proportional to the number of individuals in the population at the beginning of the breeding season. When young ones are added to the population only at specific times of the year, during well defined reproductive periods, the population is said to have geometric growth.
When populations increase periodically, the growth rate is most conveniently expressed as the ratio of the population in one year, N(1), to that in the preceding year, N(0). This ratio is expressed by the symbol λ, called the geometric growth rate. Therefore, λ = N(1)/N(0). This may be compared to λ = er as used in exponential population growth. In both the cases, X represents a factor of population increase. To project the growth of a population, the initial number, N(0), is multiplied by the geometric growth rate X once for each unit of time passed. Hence,
N(t) = N(0) λt
It may be noted that this equation for geometric growth is identical to that for exponential growth (Nt = N0ert ) mentioned above except that , λ ( the geometric growth rate) takes the place of er, the amount of exponential growth during each unit of time. This is well known that increasing populations have positive exponential growth rates and geometric growth rates greater than 1; decreasing populations have negative exponential growth rates and geometric growth rates less than 1 but greater than 0 as a real population cannot have a negative number of individuals.